Principle of film thickness measurement by spectroscopic reflectometry
Parameter fitting
There are several methods of film thickness measurement based on spectroscopic reflectometry, including the parameter fitting method and the peak/valley method. We discuss the parameter fitting method here.
Interference by thin film
When a light beam is incident on a transparent film, a portion of the beam gets reflected at the surface, but the remaining portion goes through and hits the boundary with the substrate underneath. A portion of this remaining beam gets reflected there and goes back towards the surface of the film. When the two reflected beams - one from the surface and the other from the boundary - meet, they cause interference. This is thin film interference. In reality, what actually happens is much more complex. Some portion of the reflected beam from the boundary does not go through the surface. It gets reflected by the film surface and goes back towards the boundary. It gets reflected by the boundary again, and the same phenomenon is repeated many times. Thin film interference is produced by the superposion of multiple beams.
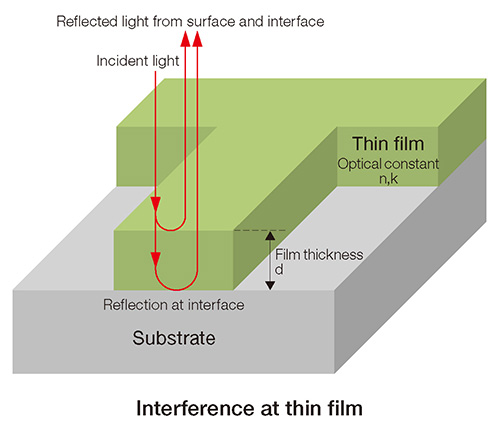
Absolute reflectance
The absolute reflectance of a material is the ratio between the intensity of reflected light from its surface and the intensity of incident light. If there is no film on the material and the incident light is normal to the surface, the absolute reflectance is determined by the Fresnel index of the surface. If, however, there is a transparent film covering the material, the absolute reflectance is determined by three parameters, that is, the Fresnel index of the film surface, the Fresnel index of the boundary between the film and the substrate, and film thickness. The Fresnel index of the surface is determined by the optical constants (refractive index and extinction coefficient) of air and those of the film. Likewise, the Fresnel index of the boundary is determined by the optical constants of the film and those of the substrate.
Simulation of absolute reflectance
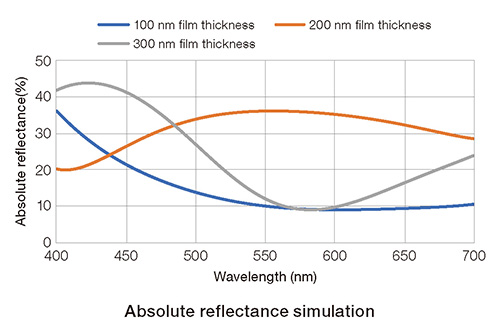
The absolute reflectance of thin film interference is calculated using the following parameters: wavelength of incident light, film thickness, optical constants of the film, optical constants of the substrate.
The figure below shows an example of simulation of absolute reflectance for a single-layer film. In this example, a reflectance spectrum is calculated for the film with three different values of thickness (100nm, 200nm, and 300nm). The same optical constants are applied to obtain all three reflectance spectra. You can see that the reflectance spectrum changes with thickness.
Film thickness measurement by spectroscopic reflectometry
From the standpoint of optical measurement, the parameters used in the simulation above are considered as follows.
Wavelength of incident light, optical constants of film, and optical constants of substrate are known parameters. Absolute reflectance is a parameter optically measurable using reflectance standards.
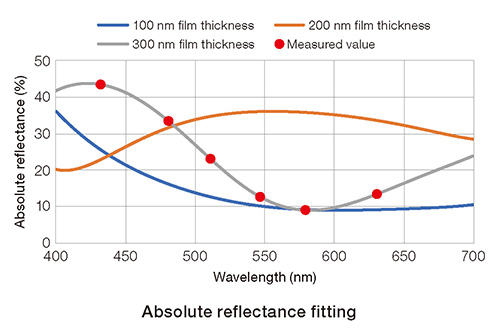
Suppose you have a film with unknown thickness. You can calculate the thickness by fitting actual measurement data with simulation. In the example below, measurement data for a film are fitted with its simulation data for thickness varying from 0nm to 350nm at 1nm intervals. The measurement data fits the simulation of 300nm thickness. Parameter fitting is an effective method when the film thickness is less than 1μm.
You might also be interested in





